NCERT Solutions for Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry
NCERT Solutions Class 9 Maths Chapter 5 – Free PDF Download
According to the Revised CBSE Syllabus 2020-21, this chapter has been removed.
NCERT Solutions for Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry are provided here for the students to refer. Euclid’s Geometry is a fundamental concept that forms the basis for much more advanced topics. Therefore, one of the guides to help you understand this concept is NCERT Solutions for Class 9 Maths Chapter 5 – Introduction to Euclid’s Geometry. It is designed by knowledgeable teachers with years of relevant experience. NCERT Solutions is one of the best guides you could adapt for your study needs.
Furthermore, we also update content as prescribed by CBSE. Moreover, solutions are drafted in a way that prioritizes ease-of-learning.Chapter 5 Introduction to Euclid’s Geometry
Download PDF of NCERT Solutions for Class 9 Maths Chapter 5 – Introduction to Euclid’s Geometry





List of Exercises in class 9 Maths Chapter 5
Exercise 5.1 Solutions – 7 Questions
Exercise 5.2 Solutions – 2 Questions
Access Answers of Science NCERT Class 9 Maths Chapter 5 – Introduction to Euclid’s Geometry
Exercise 5.1 Page: 85
1. Which of the following statements are true and which are false? Give reasons for your answers.
(i) Only one line can pass through a single point.
(ii) There are an infinite number of lines which pass through two distinct points.
(iii) A terminated line can be produced indefinitely on both the sides.
(iv) If two circles are equal, then their radii are equal.
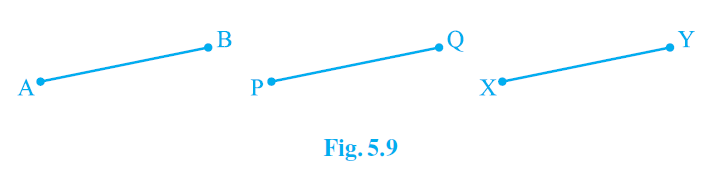
(v) In Fig. 5.9, if AB = PQ and PQ = XY, then AB = XY.
Solution:
(i) False
There can be infinite number of lines that can be drawn through a single point. Hence, the statement mentioned is False
(ii) False
Through two distinct points there can be only one line that can be drawn. Hence, the statement mentioned is False
(iii) True
A line that is terminated can be indefinitely produced on both sides as a line can be extended on both its sides infinitely. Hence, the statement mentioned is True.
(iv) True
The radii of two circles are equal when the two circles are equal. The circumference and the centre of both the circles coincide; and thus, the radius of the two circles should be equal. Hence, the statement mentioned is True.
(v) True
According to Euclid’s 1st axiom- “Things which are equal to the same thing are also equal to one another”. Hence, the statement mentioned is True.
2. Give a definition for each of the following terms. Are there other terms that need to be defined first? What are they, and how might you define them?
(i) parallel lines
(ii) perpendicular lines
(iii) line segment
(iv) radius of a circle
(v) square
Solution:
Yes, there are other terms which need to be defined first, they are:
Plane: Flat surfaces in which geometric figures can be drawn are known are plane. A plane surface is a surface which lies evenly with the straight lines on itself.
Point: A dimensionless dot which is drawn on a plane surface is known as point. A point is that which has no part.
Line: A collection of points that has only length and no breadth is known as a line. And it can be extended on both directions. A line is breadth-less length.
(i) Parallel lines – Parallel lines are those lines which never intersect each other and are always at a constant distance perpendicular to each other. Parallel lines can be two or more lines.
(ii) Perpendicular lines – Perpendicular lines are those lines which intersect each other in a plane at right angles then the lines are said to be perpendicular to each other.
(iii) Line Segment – When a line cannot be extended any further because of its two end points then the line is known as a line segment. A line segment has 2 end points.
(iv) Radius of circle – A radius of a circle is the line from any point on the circumference of the circle to the center of the circle.
(v) Square – A quadrilateral in which all the four sides are said to be equal and each of its internal angle is right angles is called square.
3. Consider two ‘postulates’ given below:
(i) Given any two distinct points A and B, there exists a third point C which is in between A and B.
(ii) There exist at least three points that are not on the same line.
Do these postulates contain any undefined terms? Are these postulates consistent? Do they follow from Euclid’s postulates? Explain.
Solution:
Yes, these postulates contain undefined terms. Undefined terms in the postulates are:
– There are many points that lie in a plane. But, in the postulates given here, the position of the point C is not given, as of whether it lies on the line segment joining AB or not.
– On top of that, there is no information about whether the points are in same plane or not.
And
Yes, these postulates are consistent when we deal with these two situations:
– Point C is lying on the line segment AB in between A and B.
– Point C does not lie on the line segment AB.
No, they don’t follow from Euclid’s postulates. They follow the axioms.
4. If a point C lies between two points A and B such that AC = BC, then prove that AC = ½ AB. Explain by drawing the figure.
Solution:

Given that, AC = BC
Now, adding AC both sides.
L.H.S+AC = R.H.S+AC
AC+AC = BC+AC
2AC = BC+AC
We know that, BC+AC = AB (as it coincides with line segment AB)
∴ 2 AC = AB (If equals are added to equals, the wholes are equal.)
⇒ AC = (½)AB.
5. In Question 4, point C is called a mid-point of line segment AB. Prove that every line segment has one and only one mid-point.
Solution:

Let, AB be the line segment
Assume that points P and Q are the two different mid points of AB.
Now,
∴ P and Q are midpoints of AB.
Therefore,
AP = PB and AQ = QB.
also,
PB+AP = AB (as it coincides with line segment AB)
Similarly, QB+AQ = AB.
Now,
Adding AP to the L.H.S and R.H.S of the equation AP = PB
We get, AP+AP = PB+AP (If equals are added to equals, the wholes are equal.)
⇒ 2AP = AB — (i)
Similarly,
2 AQ = AB — (ii)
From (i) and (ii), Since R.H.S are same, we equate the L.H.S
2 AP = 2 AQ (Things which are equal to the same thing are equal to one another.)
⇒ AP = AQ (Things which are double of the same things are equal to one another.)
Thus, we conclude that P and Q are the same points.
This contradicts our assumption that P and Q are two different mid points of AB.
Thus, it is proved that every line segment has one and only one mid-point.
Hence Proved.
6. In Fig. 5.10, if AC = BD, then prove that AB = CD.
Solution:

It is given, AC = BD
From the given figure, we get,
AC = AB+BC
BD = BC+CD
⇒ AB+BC = BC+CD [AC = BD, given]
We know that, according to Euclid’s axiom, when equals are subtracted from equals, remainders are also equal.
Subtracting BC from the L.H.S and R.H.S of the equation AB+BC = BC+CD, we get,
AB+BC-BC = BC+CD-BC
AB = CD
Hence Proved.
7. Why is Axiom 5, in the list of Euclid’s axioms, considered a ‘universal truth’? (Note that the question is not about the fifth postulate.)
Solution:
Axiom 5: The whole is always greater than the part.
For Example: A cake. When it is whole or complete, assume that it measures 2 pounds but when a part from it is taken out and measured, its weigh will be smaller than the previous measurement. So, the fifth axiom of Euclid is true for all the materials in the universe. Hence, Axiom 5, in the list of Euclid’s axioms, is considered a ‘universal truth.
Exercise 5.2 Page: 88
1. How would you rewrite Euclid’s fifth postulate so that it would be easier to understand?
Solution:
Euclid’s fifth postulate: If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely, meet on that side on which the sum of angles is less than two right angles.
i.e., the Euclid’s fifth postulate is about parallel lines.
Parallel lines are the lines which do not intersect each other ever and are always at a constant perpendicular distance apart from each other. Parallel lines can be two or more lines.
A: If X does not lie on the line A then we can draw a line through X which will be parallel to that of the line A.
B: There can be only one line that can be drawn through the point X which is parallel to the line A.
2. Does Euclid’s fifth postulate imply the existence of parallel lines? Explain.
Solution:
Yes, Euclid’s fifth postulate does imply the existence of the parallel lines.
If the sum of the interior angles is equal to the sum of the right angles, then the two lines will not meet each other at any given point, hence making them parallel to each other.

∠1+∠3 = 180o
Or ∠3+∠4 = 180o
NCERT Solutions for Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry
Chapter 5 Introduction to Euclid’s Geometry belongs to Unit 4: Geometry. This particular unit carries 28 marks out of 100. Therefore, it is quite important to ensure that this chapter is studied thoroughly. The important topics that are covered under this chapter are:
- Euclid’s Definitions
- Axioms and Postulates
Euclidean Geometry is a system introduced by the Alexandrian-Greek Mathematician Euclid around 300 BC. More than 2,000 years later, the contributions of Euclid still remain valid. It has practical applications in several fields, ranging from engineering to theoretical physics. It even has academic significance and implications in various disciplines of mathematics and science.
Explore how Euclidean Geometry works and discover the various theorems. Find more important NCERT Solutions For Class 9 Maths to help you practise.
Key Features of NCERT Solutions for Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry
- Elaborate and detailed content
- Formulas are highlighted
- Explanations are presented in an easy-to-understand language
- Designed by qualified teachers
- Includes latest questions from the prescribed syllabus
- Extensive analysis of previous year question papers
- Explore additional learning resources such as sample papers and more
Frequently Asked Questions on NCERT Solutions for Class 9 Maths Chapter 5
Is NCERT Solutions for Class 9 Maths Chapter 5 suitable for CBSE students?
NCERT Solutions have been prescribed for years as a complete source of information to CBSE students, to develop their analytical skills. They have proven to be essential for learning the syllabus and developing the confidence that is required to face their exams. The NCERT Solutions for Class 9 Maths Chapter 5 explain the steps with precision, without missing out on essential aspects of solving a question.
Where can I download NCERT Solutions for Class 9 Maths Chapter 5?
NCERT Solutions for Class 9 Maths Chapter 5 Statistics are provided in BYJU’S website, which is considered to be one of the most important study materials for students studying in Class 9. The solutions provided at BYJU’S are formulated in such a way that every step is explained clearly and in detail. The Solutions for Class 9 Maths NCERT are prepared by the subject experts to help students in their board exam preparation. It is very important for the students to get well versed with these solutions to get a good score in the Class 9 examination.
What is the meaning of Euclidean geometry according to NCERT Solutions for Class 9 Maths Chapter 5?
Euclidean geometry is the study of geometrical shapes and figures based on different axioms and theorems. It is basically introduced for flat surfaces. It is better explained especially for the shapes of geometrical figures and planes. By referring to NCERT Solutions for Class 9 Maths Chapter 5 students can score well in exams.